
The box-and-whisker plot is a bit more complex than the other chart types. For running backs (RB), the typical speed for this position in the 2014 draft was 4.57 seconds. The upper whisker is another name for the maximum, and the lower whisker is another name for the minimum. Let’s click on one of the box-and-whiskers for more information:Ĭlicking on one will bring up a tooltip with the different data points defined.

That is why that data point is an outlier. If the bottom whisker extended down to either one of those outliers, the lower whisker would be significantly longer than the upper whisker (or quartile). the faster players), you can see that they are approximately the same size for those two positions. the slower players) and the compare it to the lower whisker (i.e. In the guards (OG) and tight ends (TE), you can see the only two outliers on the plot. You can see the standard grouping of speed by each position in the box and the dispersion of widening scores in the whiskers. The visualization is a representation of the 40-yard dash times from the 2014 NFL Scouting Combine broken out by position.

The plot above (Figure 2) is a great way to illustrate the effectiveness of the box-and-whisker plot. Instead, it is an outlier, which is represented by a single dot. For instance, what if the data set above had another number in the range like 287? That’s so far out of the normal set of data that it wouldn’t make sense to let it serve as the maximum. Things get a little more complicated when you start to consider outliers or data points that are not representative of the data set. The median of the upper half or third quartile The median of the lower half or first quartile We’ll illustrate how a minimum, Q 1, the median, Q 3, and the maximum are derived from them. That forms the “whiskers” of the plot.Ĭonsider the numbers above as a very simple data set. That forms the “box.” The maximum of the data set is the upper range while the minimum of the data set is the lower range. These are simply the median of the upper half of the data and the median of the lower half of the data. Then, the upper and lower quartiles are determined.
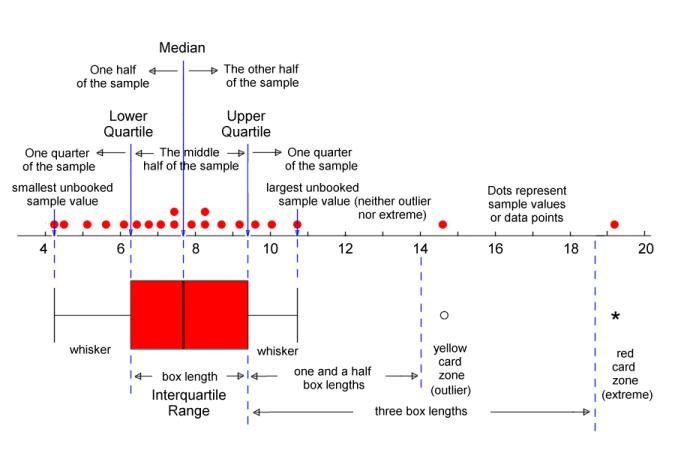
That is where the box turns from grey to light grey. You start by determining the median of the data set. Each quartile has a specific numeric value, determined from the data set. The whiskers represent the distances between the lowest value to the first quartile and the fourth quartile to the highest value. The box represents the values between the first and third quartile (also known as the interquartile range=Q 3 – Q 1).
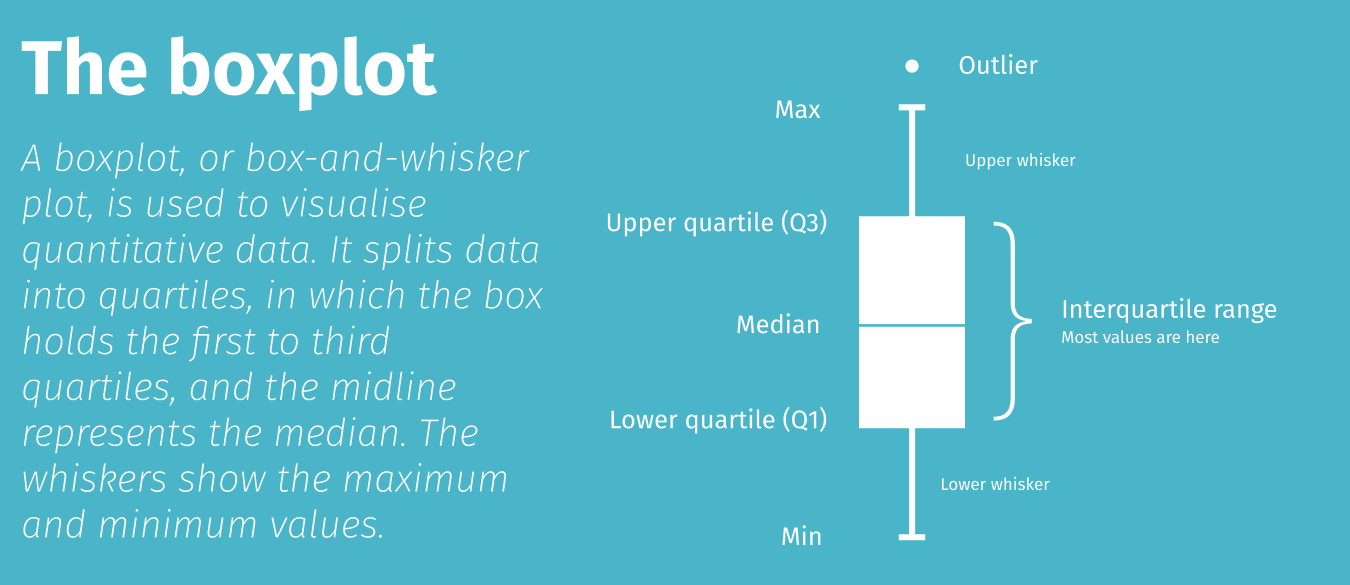
Before we can delve into an example of how it is used within Tableau Software, we have to explain the logic behind this type of view.
How to read a box and whisker plot series#
Since there are so many cool features to cover in Tableau, the series will include several different posts.Ĭompared to the other chart types, the box-and-whisker plot (also known as the box plot) is a bit more complicated. The series is intended to be an easy-to-read reference on the basics of using Tableau Software, particularly Tableau Desktop. To help Tableau rookies, we’re starting from square one with the Tableau Essentials blog series. Not everyone is a Tableau guru, at least not yet.


 0 kommentar(er)
0 kommentar(er)
